How To Find Equilibrium Points Of A System Of Differential Equations
How to find equilibrium points of a system of differential equations. D x d t 3 x 2 y. In a sense this system therefore has an equilibrium point at 0. Given a slope field you can find equilibrium solutions by finding everywhere a horizontal line fits into the slope field.
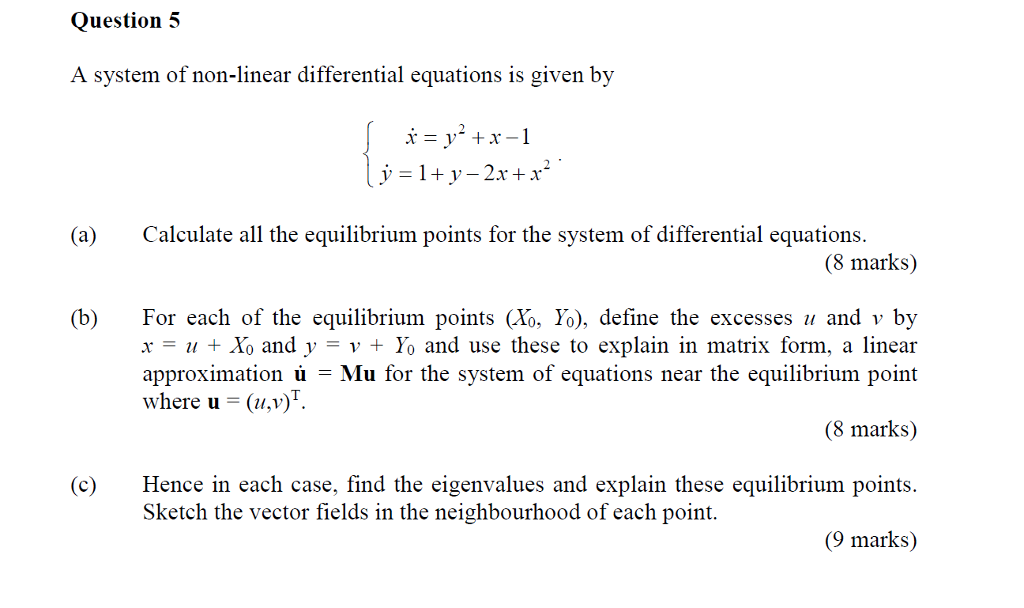
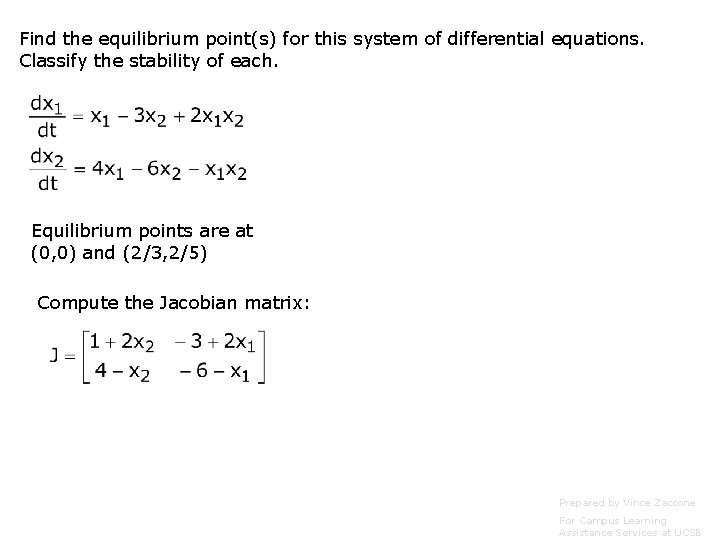
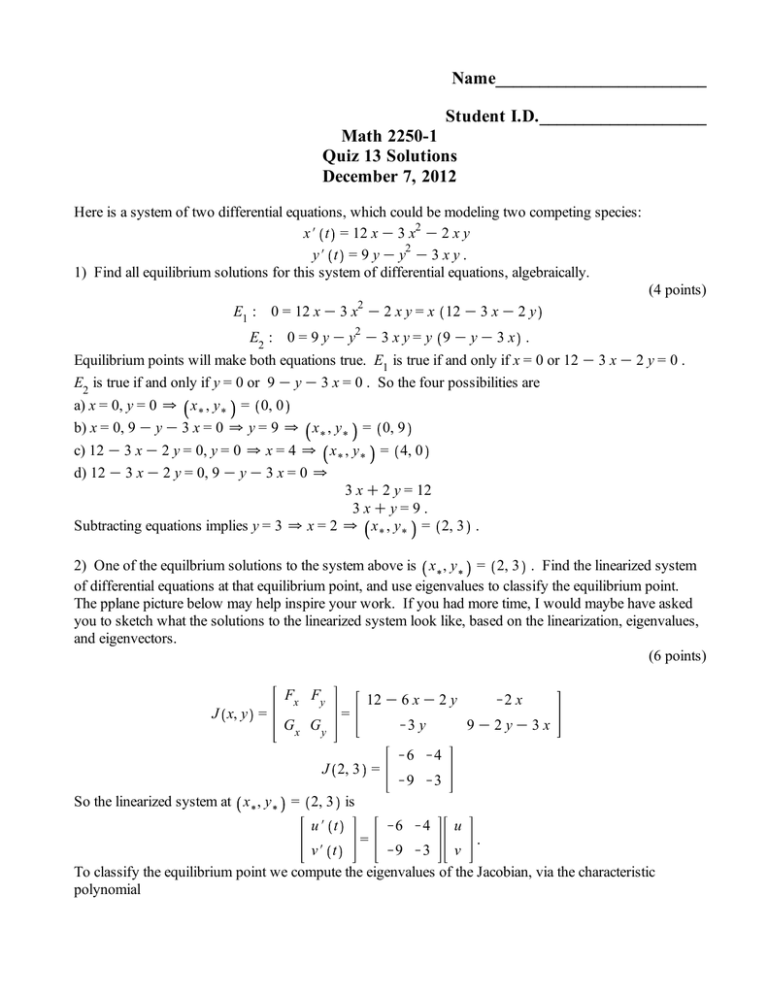
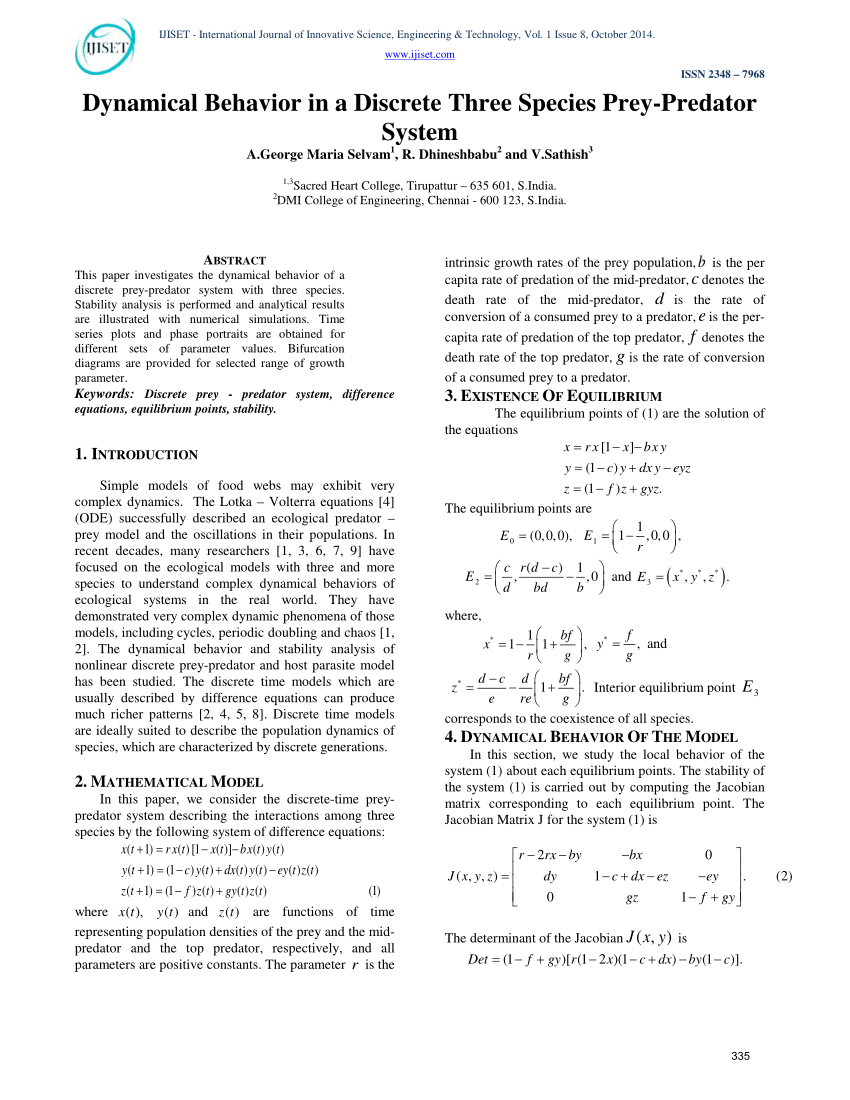
Linearization of Differential Equation Models 1 Motivation We cannot solve most nonlinear models so we often instead try to get an overall feel for the way the model behaves. Texdotxx-ytex texdoty1-extex The equilibrium points satisfy the system of algebric equations. Dx1 dt dx2 dt Analytical approach solve system of non-linear equations to find equilibria if needed compute Jacobi.
Dy dx 5x3y dy dx 6x2y d y d x 5 x 3. An equilibrium solution is a solution to a DE whose derivative is zero everywhere. Use scipyoptimizefsolve to find them if you know good starting points.
Dx dt 2x 1 x 2 xy dy dt 4y 1 y 4 3xy. In the case of a singular matrix the system has an infinite number of equilibrium points. These four solutions are called separatrix.
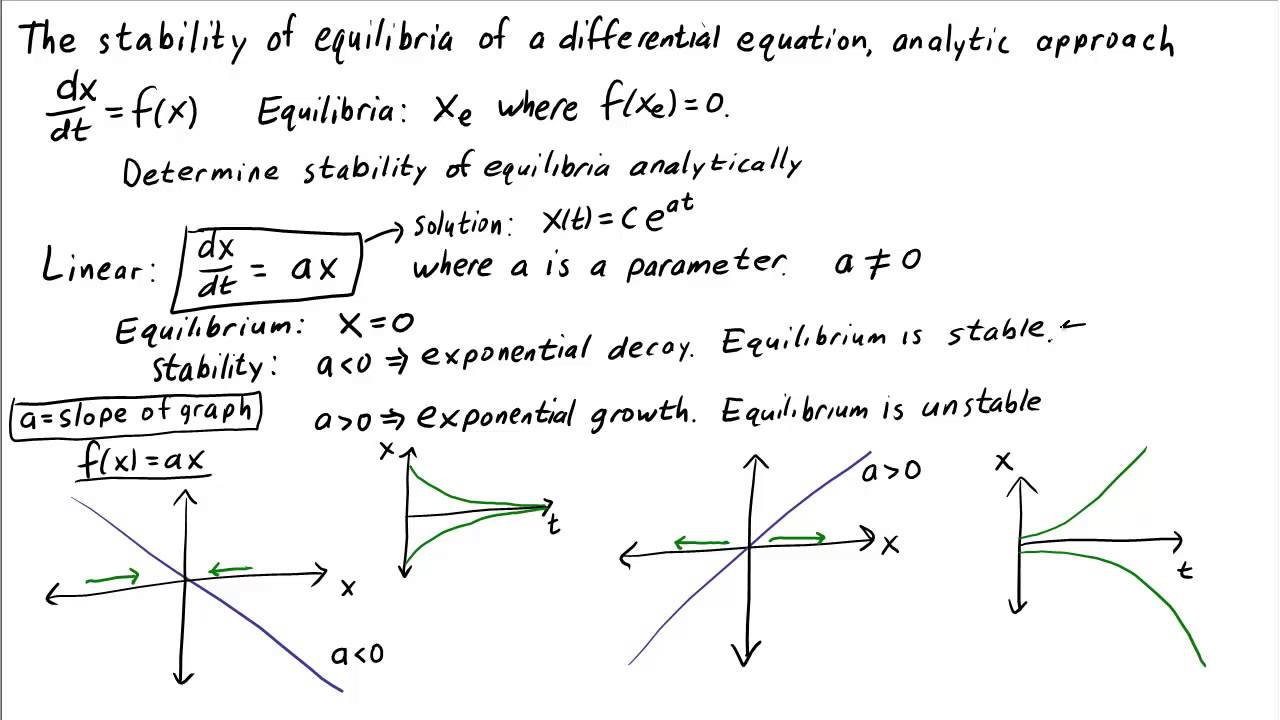
As with the single differential equations case equilibrium solutions are those solutions for which. Tag4 From 4 we have x2 y2. X fx u 0 Result is an algebraic set of equations that must be solved for both x e and u e Note that x e 0 and u e 0 by definition.
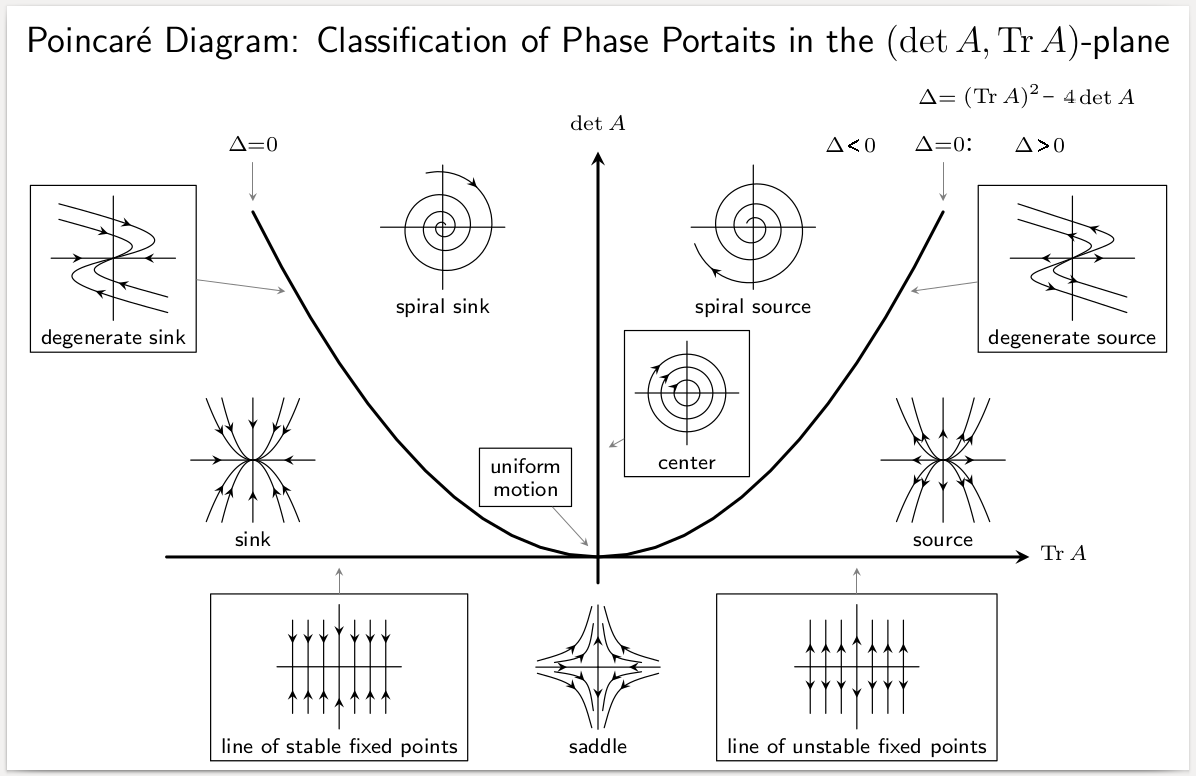
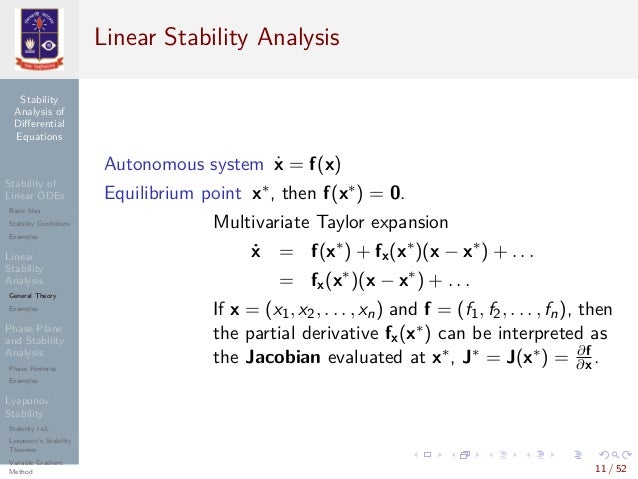
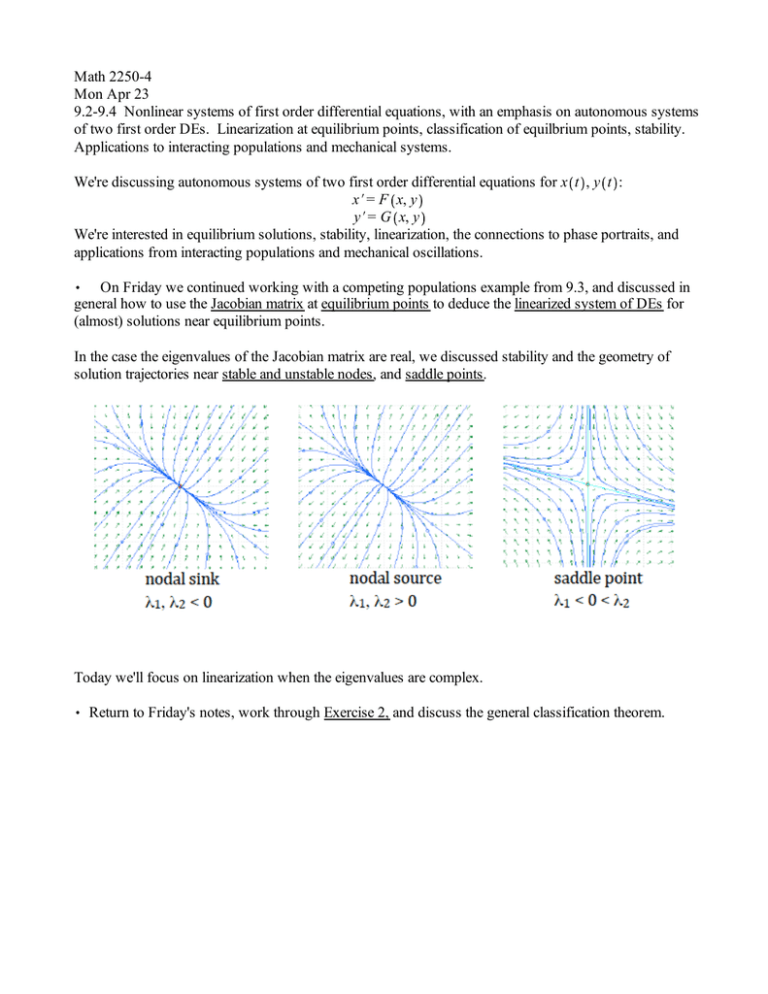
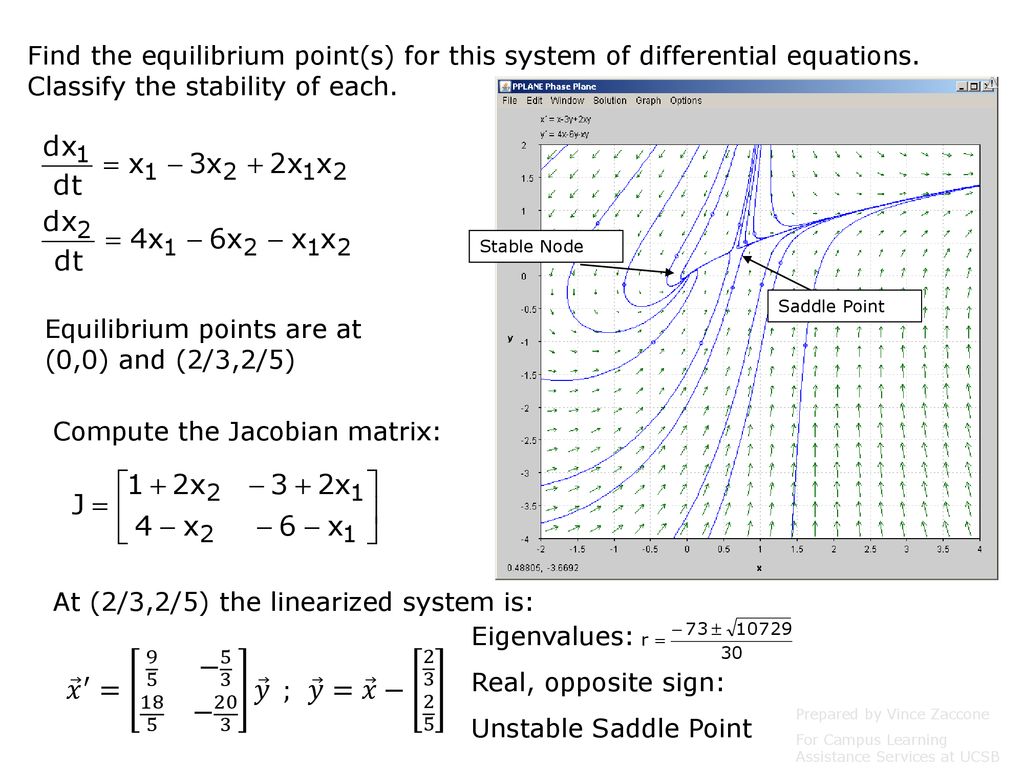
Classification of equilibrium points is determined by the eigenvalueslambda _1lambda _2 of the matrix A The numbers lambda _1lambda _2 can be found by solving the auxiliary equation. Example 811 The following system of three equations the so-called Lorenz system arose as a crude model of uid motion in a vessel of. All I know if Im even correct is that we need to make sure that d.
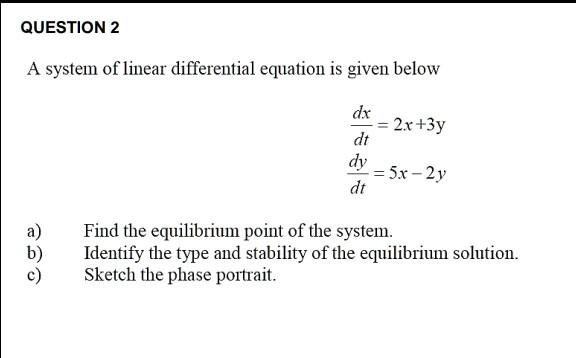
D y d t 6 x y. 2 Find the nullclines of the system.
This leads us to a very important theorem.
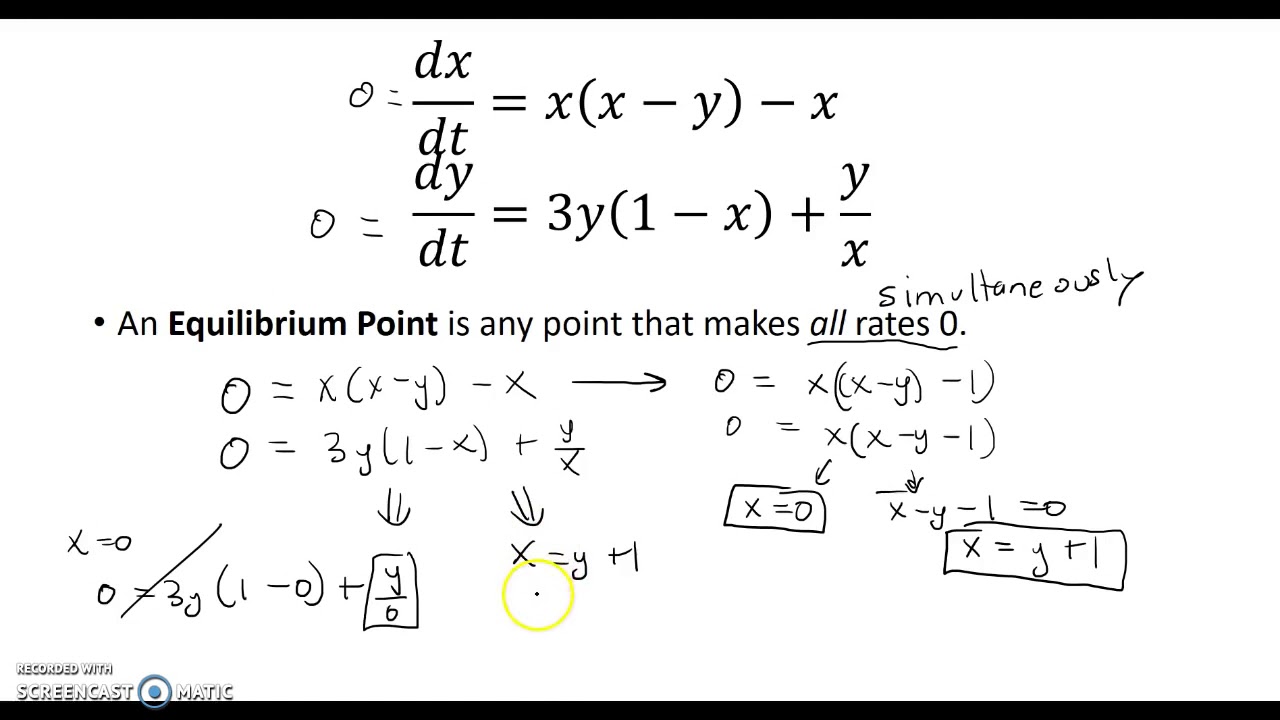
These equilibrium positions will be stable because lambda_2 -2 lt 0 Combining the results we can write the final answer. Example 13 Consider the differential equation dx dt x 1 t 1 t2. The equilibrium point is unstable if at least one of the eigenvalues has a positive real part. Texdotxx-ytex texdoty1-extex The equilibrium points satisfy the system of algebric equations. This leads us to a very important theorem. The point is an equilibrium point for the differential equation d x d t f t x displaystyle frac dmathbf x dtmathbf f tmathbf x if f t x 0 displaystyle mathbf f ttilde mathbf x mathbf 0 for all t displaystyle t. We are given a system of differential equations. As RobertLewis has pointed out we find the equilibrium points xy at the points where we have x y 0. It follows that we eventually get solutions which exponentially decay to zero.
Differential Equations Equilibrium points and Stable points - YouTube. Texx-y0tex tex1-ex0tex Which means you have only 00 as an equilibrium point. Find all equilibria of the following system of differential equations and use the analytical approach to determine the stability of each equilibrium. For the linear system theses solutions are lines but for the nonlinear system they are not in general. In fact what were doing here is simply an extension of this idea to systems of differential equations. Example 13 Consider the differential equation dx dt x 1 t 1 t2. For a linear system of equations the origin is always an equilibrium point though there.







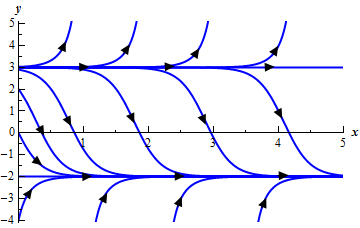

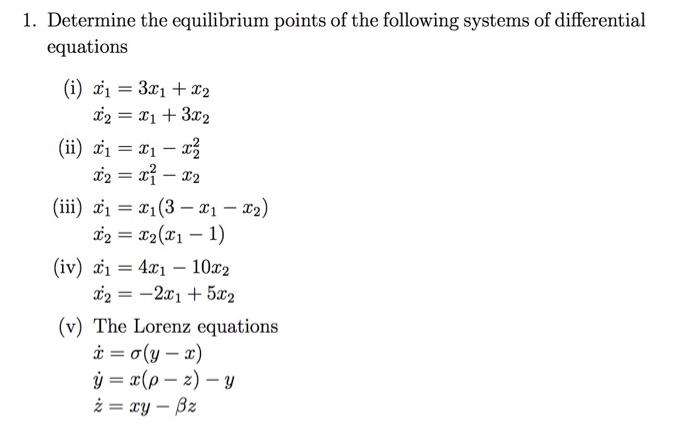




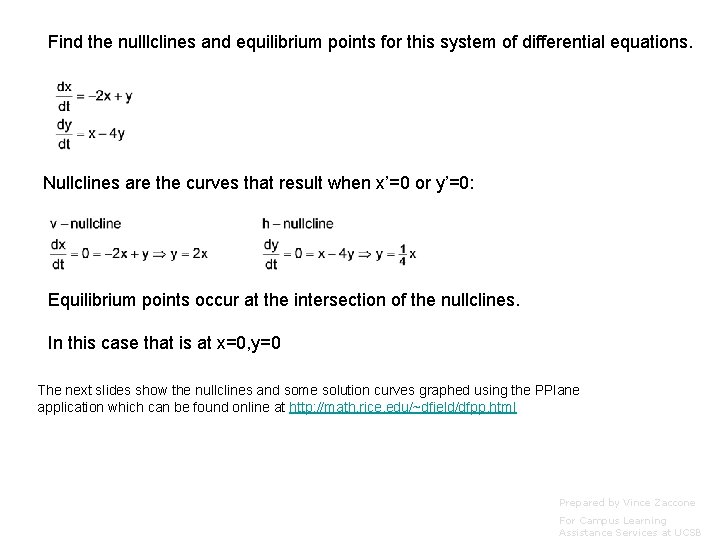

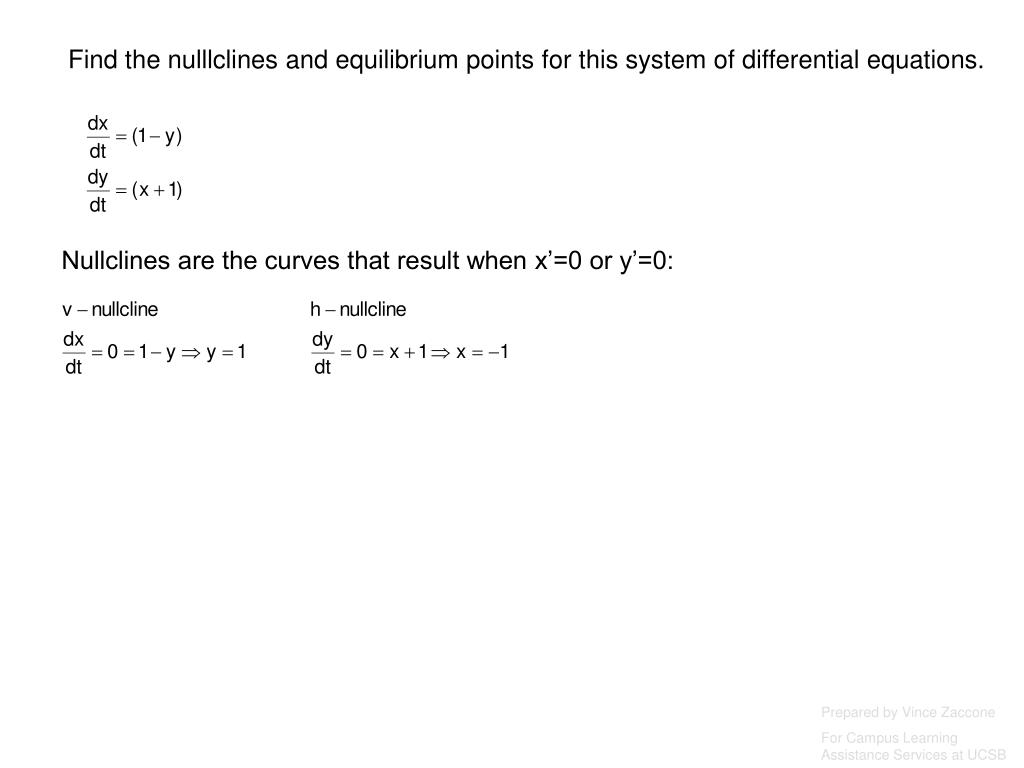











Post a Comment for "How To Find Equilibrium Points Of A System Of Differential Equations"